Variations in Gamma

The conditions for an eclipse

We found right at the start of the main page that two conditions must be satisfied before an alignment of Sun, Moon and Earth, and therefore an eclipse, can occur. The first is reasonably obvious - the Moon must be New (else it will be nowhere near the correct position). The second is not so straightforward, and comes about because the plane of the Moon's orbit round the Earth is not exactly in the plane of the Earth's orbit round the Sun - it's tilted by about 5 degrees in fact. This means that, for most New Moons, as seen from the Sun the Moon is either slightly above the Earth or slightly below and so there's no eclipse. However, if a New Moon should occur when the Moon is at one of the two points in its orbit when it is passing through the plane of the Earth's orbit (called "nodes") then it will indeed be directly between the Sun and the Earth, resulting in an eclipse.

In the diagrams below, we have the Moon orbiting the Earth which is in turn orbiting the Sun - if we assume the viewpoint is from above the Sun's north pole then both bodies orbit their parent in an anticlockwise direction. As the Moon's orbit is tilted with respect to the plane of the Earth's orbit, part of it (the light-green bit) will be above the plane and part (the dark-green dashed bit) will be below. The nodes, the points where the Moon's orbit cuts the plane of the Earth's orbit, are labelled A (for ascending) and D (for descending): so called because the Moon will be rising above the plane at the ascending node and dropping below it at the descending node. The black line joining them is called the "line of nodes". The smaller diagram gives the view from the Sun along the Sun-Earth line, and is to scale with the view from above the Sun's pole. It is clear in this view how half the Moon's orbit is above the plane and half below it.

When the line of nodes is not aligned with the Sun-Earth line, as here, it is clear that the Moon will pass below the Earth when it moves between the Earth and the Sun i.e. at New Moon. There can thus be no eclipse. The same conclusion would hold if the Moon had just passed the ascending node, only this time the Moon would be above the Earth when New.	Only when the line of nodes points along the Sun-Earth line will the Moon pass directly between the Earth and the Sun at New Moon, producing an eclipse (labelled E and shown in pink).

Alignments at a central eclipse

So far, so good. Let's now zoom in on the diagram at bottom right just above, to give the one below. I've reduced the tilt of the Moon's orbit, which was shown much larger than it actually is for reasons of clarity, but even so it is still more than the true figure of 5 degrees. I've also shown the Moon at the point of eclipse rather than at an arbitrary point on its orbit.

Here then we have a diagram of a perfectly aligned solar eclipse i.e. one with gamma equal to zero, as seen from the Sun. The question now is - what would the diagram look like one Saros later?

To answer this question we must consider the definition of the Saros Period i.e. the time between successive eclipses in a Saros Series. This is equal to 223 synodic (i.e. New Moon to New Moon) months or 242 (i.e. Node to Node) months, which [using values appropriate for 2000AD] come to 6585.3213 days and 6585.3574 days respectively. While these numbers are exceedingly close (thus accounting for the very similar characteristics of each eclipse in a Series), they are not quite the same: there is a difference of 0.0361 days (or 51 minutes 59.04 seconds). In other words, after one "synodic Saros", the Moon will be exactly New again but it (and of course the Earth) will not be exactly aligned with the node again as the "draconic Saros" is fractionally longer. Which raises another question - we know that the node will not sit at the centre of the Earth this time, but will it be to the left or to the right? This will be determined by how the line of nodes moves with respect to the orbit of the Earth.

The line of nodes

In fact, to a good approximation, the line of nodes maintains a fixed position in space. A little thought should convince you that, therefore, the line of nodes will align with the Earth-Sun line every 6 months. Also, when viewed from above, the line will rotate in a clockwise direction relative to the Earth, as the Earth goes round the Sun in an anti-clockwise direction. Therefore, when viewed from the Sun, the node which is about to be involved in an eclipse will move from right to left. This is probably the hardest part of the whole explanation to visualise, so it might help to refer to the top-right diagram of those shown initially. Imagine that the line of nodes continues to point "up and down the page" while the Earth moves anti-clockwise round its orbit. The next time the line of nodes will again point directly along the Earth-Sun line will be when the Earth has reached the "six o'clock" position i.e. after 6 months. Also, when viewed from the Sun, while the lower part of the line of nodes is pointing straight at the Sun when the Earth is at the "twelve o'clock" position, when the Earth has reached "nine o'clock" (it's going anti-clockwise, remember) the line is pointing 90 degrees to the left i.e. it has moved from right to left. Therefore, when the Earth is approaching "six o'clock", and heading for another eclipse, the node which is about to be involved (which is, in fact, the opposite node from the previous eclipse) will approach the "eclipse zone" from the right.

As I said, this is the bit which requires one to have a good sense of spatial geometry so I hope that if you think about it long enough you'll eventually persuade yourself of the above conclusions. Of course if you've caught on immediately and are thus wondering why I'm making so much of a fuss about it I can only say "well done"!

Alignments at an "off-centre" eclipse

However, we now have all the information we need to answer the "left or right" question. We know that the node will be approaching the eclipse zone from the right, and that it will not quite have got into position by the time the Moon is New. Therefore, the node must be to the right of the Earth-Sun line at the time of the eclipse. This is shown by the next diagram:-

Here we have the situation one Saros Period after the exactly central eclipse, with the node to the right of the Earth-Sun line as it has not yet had enough time to come into exact alignment. Given that the position of the Moon must be somewhere on the green line marking its orbit, if the node is to the right of the Earth-Sun line the Moon must clearly be above the centre of the Earth at eclipse-time, as shown, as this is where the extension of the green line backwards from the node takes us.

If we now consider the position one Saros Period before the central eclipse, it should be clear that the node will this time be to the left of the Earth-Sun line, given that it must be exactly on the line one Period later. This is shown by the next diagram:-

Here we have the situation one Saros Period before the exactly central eclipse, with the node to the left of the Earth-Sun line. Again, as the position of the Moon must be somewhere on the green line marking its orbit, if the node is to the left of the Earth-Sun line the Moon must clearly be below the centre of the Earth at eclipse-time, as shown, as this is where the extension of the green line (this time forwards from the node) takes us.

[Note that, for clarity, I have much exaggerated the extent to which the node fails to align before or after one Saros Period]

So, putting everything together, we can see that for Saros Series whose eclipses are, as in the case of those above, at the descending node (even-numbered Series, in fact), the first eclipses will occur at the south of the Earth and then move steadily northwards, to cross the mid-line and finally end at the north.

Eclipses at the ascending node

I hope it is clear that for Saros Series whose eclipses are at the ascending node (odd-numbered ones) the situation is exactly reversed from that at the descending node: eclipses start at the north and move south. This is because it makes no difference whether one is considering the ascending or descending node: they both approach the eclipse zone "from the right". Therefore, all the diagrams above are just "turned upside down" in the case of the ascending node, as shown below.

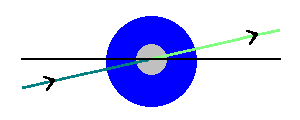Eclipse at the ascending node, before the central eclipse.	Eclipse at the ascending node, the central eclipse.	Eclipse at the ascending node, after the central eclipse.

Epilogue

The above constructions explain why the eclipses in even-numbered Saros Series begin in the south and move steadily north while those in odd-numbered Saros Series begin in the north and move steadily south. There is, however, one "loose end" to tie up. This refers to my comment that the line of nodes maintains a fixed position in space "to a good approximation". In fact, as I have already mentioned on the main page, due to the way the gravitational attraction of the Sun affects the Moon's orbit, the line actually rotates clockwise, making one turn every 18.61 years. This does not materially affect any of the conclusions above but does mean that the line of nodes in fact aligns with the Earth-Sun line every 173.31 days rather than 182.62 (i.e. 6 months) - the true period is shorter because the line rotates in the same direction as its movement relative to the Earth.