The (theoretical) interval between successive conjunctions is given by the Jupiter-Saturn synodic period, which is equal to 19.859 years. I say "theoretical" because the synodic period relates to the view from the Sun, not the Earth. The fact that the view from the Earth is often at a different angle to that from the Sun does affect things somewhat, and can cause differences of a few months between the theoretical and actual conjunction dates. The Earth's motion also causes what are known as triple conjunctions - occasions when Jupiter and Saturn are close together three times in a relatively short space of time. These occur when the planets are at opposition (directly opposite the Sun as seen from Earth), because with this geometry the motion of the Earth as it overtakes the planets causes them (in particular Jupiter, being nearer) to describe a zig-zag pattern in the sky which brings them close together three times rather than just one. The most recent triple conjunction was in January, March & July 1981 but the conjunctions were not close ones - about 1 degree. The next is not until 2238/9.
Because the orbits of the two giant planets are slightly inclined with respect to each other and that of the Earth, the visual separation between the two at conjunction can vary considerably. At worst it can be as much as 1.3 degrees (almost three times the width of the Moon), but this is still close by the standards of most planetary conjunctions as can be seen from my main "Conjunctions" page. Importantly though, when they meet near to one of the two points where their orbits seem to cross as seen from the Earth a very close conjunction can occur. It is difficult to give an accurate estimate of what "near" means because the separation as well as the timing is affected by the line of sight from the Earth, which depends on its position on its orbit. However, a conjunction occurring at about 10 degrees or less from a crossing point will generally result in a separation of about one-tenth of a degree. The very least separation in the period from 1200 to 2400AD was a mere one-thirtieth of a degree, in 1226. The 2020 event fell in the "very close" camp, with a separation of just 6.1 arc-minutes (where there are 60 arc-minutes in a degree). This was the closest observable event since the remarkable 1226 conjunction, as that in 1623 (at 6.0 arc-minutes) happened when the planets were too near the Sun in the sky to be visible.
Very close conjunctions tend to group in sets of three whose members are about 60 years apart, because this period (actually 59.577 years - three synodic periods) is very nearly equal to 2 full orbits of Saturn and 5 full orbits of Jupiter. So if the planets were in a favourable orientation in a given year they will be close to that same orientation 60 years before and 60 years afterwards as well. This is shown by the observation that there will be a close conjunction in 2080 and there was a reasonably close one in 1960. However, "very nearly equal" is not "equal". The number of Saturnian orbits is in fact 2.022486 rather than exactly 2.0 and this extra bit moves the conjunction point round by 8.10 degrees each cycle of 60 years. This is well within the 10 degree tolerance for a close conjunction as mentioned above and so one cycle forward or back from a near-optimum position can still result in a close conjunction. After two cycles the orientation will be quite far from optimum though, hence the sets of just three.
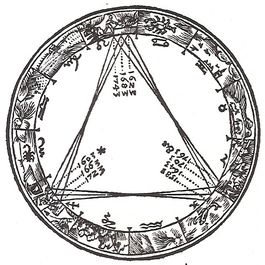
 | The interval of about 400 years between 1226 and 1623, and then between 1623 and 2020, might suggest that there is an overall periodicity to very close Jupiter-Saturn conjunctions and indeed that would be true. As mentioned above, it takes three synodic periods of 19.859 years to get the conjunction position "back to the start", which means that conjunction positions are distributed each 120 degrees around the planets' orbits - the diagram to the left, drawn by the famous astronomer Johannes Kepler, illustrates not only this but also the "set of three" effect. Note however that, as mentioned in the first paragraph, there are two positions where a close conjunction can take place, 180 degrees apart, and so if the position ahead of the optimum one moves round (due to the "and a bit" effect noted above) by just 60 degrees it will then be in the other optimum position and so a close conjunction will occur. Time for a bit more arithmetic, therefore! |
As mentioned above, each cycle of three conjunctions shifts the conjunction point by 8.10 degrees. To shift the conjunction positions round by the 60 degrees required to align with the next optimum position therefore takes 7.41 cycles. However, it only makes sense to consider complete cycles (as the planets are only in position again after a full cycle has completed) and so the periodicity would appear to be 7 times 19.859 times 3, which is 417.04 years. But remember - the conjunction position which has now become optimum is the one which was already one conjunction period ahead of the one we began with and so we must subtract one period. This gives us the true periodicity of (in round numbers) 397 years, which is indeed the interval between the close conjunctions already described. Note though that as 7.41 is only a little closer to 7 than 8 this period is not particularly well defined and things will soon "slip out of sync". We know that there is a degree of leeway around the optimum position though and so what this means in practice is that the usual 397 year periodicity will sometimes drop back by one cycle, to give an interval of 457 years. The next such occasion is the interval between the conjunctions in 2417 and 2874. Over a long-enough timescale, a mixture of 2 "long" periods to 3 "short" ones produces the average periodicity of 7.41 minus one-third cycles required to keep everything in step.
All totally clear, I trust?